One of the main reasons why dawn calculation methods such as using a fixed depression angle (i.e. 18°) can not be universally applied is that it does not take into account key considerations such as the tilt of the earth and the impact it can have on northern latitude countries such as the UK.
The tilt of the earth has a major impact of the length of the night between seasons and critically the rate of change for the depression angle of the sun. For locations closer to the equator the rate of change remains relatively constant. In Mekka, the transit of the sun between one degree takes 5 minutes and this is constant all year round. In the UK, the time for the sun to transit one degree is 6 minutes in the winter and 16 minutes at the height of summer.
The chart below illustrates the impact of the rate of change on the calculation of dawn (Subh al-Sadiq). The left axis show the sunrise and the calculated dawn time across the year. The right axis shows to the nearest minutes the time it takes for the sun to transit by one degree.
The chart shows the impact of the rate of change of the depression angle of the sun on the established time for dawn (Subh al-Sadiq). The spring and autumn equinox occurs in March and September respectively. At the equinox the length of day and night matches and so we see the smallest rate of change (6 minutes). The resulting impact on dawn is the slightly shorter 'gap' between dawn (red line) and sunrise (black line) in March and September when compared with the month before and after.
January to Mid-April and September to December are periods when the (rounded) rate of change only changes between 6 and 7 minutes During these months, the time between dawn and sunrise remaining relatively constant.
During May, the rate of change goes from 9 minutes to 14 minutes by the end of the months. When compared to the time between dawn and sunrise at the start of May and the end of May, a noticeable increase can be seen.
During the month of June which includes the shortest night (summer solstice), the rate of change reaches 16 minutes but the difference during the month is within a range of 3 minutes (14 to 16 minutes) which results in a more relative gap between dawn and sunrise but this gap being bigger than that experience in the winter months.
Like May, July sees a large change in the depression rate of change (15 down to 11 minutes). When compared with the time difference between dawn and sunrise, it is clear the longer rate of change at the start of the month results in a bigger gap between dawn and sunrise. A similar pattern can be seen in August.
[Click Image to Expand]
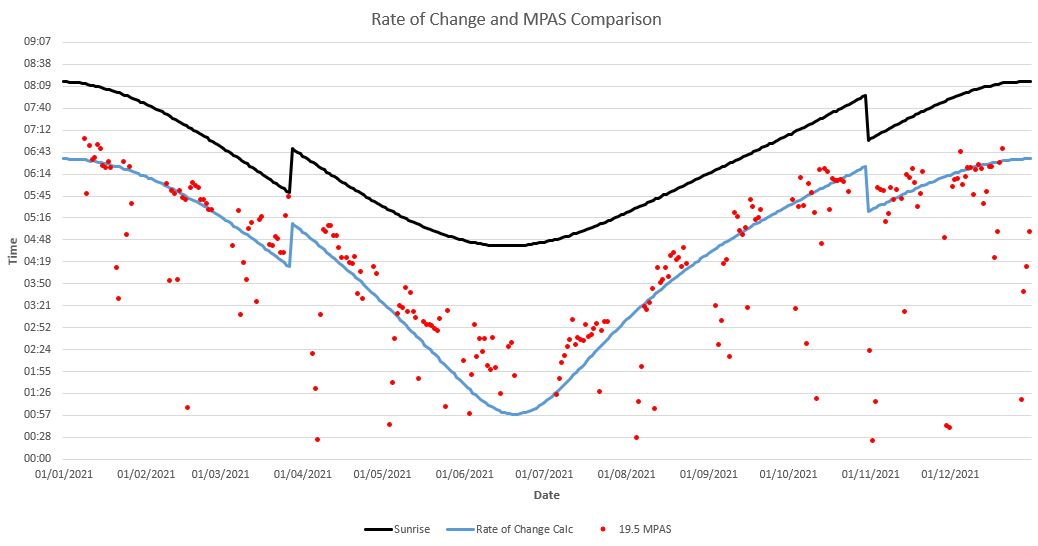
By calculating the period (in seconds) it takes the sun to travel 1° on each day and applying this to a fixed 'multiplier', it is possible to establish a dawn time will corelates to observations and the reading from the Sky Quality Meter (SQM). The following chart show the resulting 'rate change' dawn times:
[Click Image to Expand]
This relationship between the 'rate of change' calculation and MPAS readings are better illustrated when the LOWESS function is applied:
There is almost no difference between the LOWESS 19.5 MPAS result and the depression angle of 14.5° during the "Normal Period". The "Adjusted Period" shows a dawn time calculated by the LOWESS function not bound to a fixed depression angle. The separation between the two (LOWESS calculation and 14.5°) begin and ends at the "Adjusted Period" - when the sun does not go below 18°.
[Click Image to Expand]
There is almost no difference between the LOWESS 19.5 MPAS result and the 'rate of change' calculation for the majority of the year. During the summer months where the sun does not go below 18°, there is a marked difference between the two. The reasonable explanation for this difference is after the certain point the increased period (in seconds) which results from the 'rate of change' calculation does not impact on twilight. By substracting the fixed period (in seconds) established from the last day before the sun stops reaching 18° from sunrise to each day in the summer period, you get the following dawn curve:
[Click Image to Expand]
By calculating dawn as a function of 'rate of change' using a fixed 'multiplier' and freezing the time difference once you reach a sun depression angle of 18°, you are able to establish a dawn time which is very close to SQM data and naked-eye observations.
hat the rate of change analysis shows:
- The depression angle of the sun obviously determines the level of twilight
- The depression angle can not be used in isolation but the rate of the change also needs to be considered
- For the majority of the Islamic world (closer tot the equator), the rate of change remains relatively constant
- For northern latitude countries (like the UK), the rate of change does not remain constant but changes greatly
- The different rate of changes over the months and seasons has a significant impact in the resulting dawn times